The Heat is on! A huge amount of the technology and comforts that we have in our world just wouldn’t exist if it wasn’t for what we’ve learned about the way heat behaves. So keep cool and use this excellent series to teach your students everything that they need to know about heat, including its effect on things and how it transfers from one thing to another.
In Episode 4, The Kelvin Scale, we look at the temperature scale that in many ways is superior to the Celsius and Fahrenheit scales. Zero on the Kelvin scale is equal to -273°C. And why is -273°C so special? Because that’s the coldest temperature you can get!
A 3-minute excerpt.
The Episode 4 Question Sheet for Students:
The PDF version.
Google The Google Doc version. Google
Get the answers.
If you have ClickView, watch the whole episode here.
If you have Learn360, watch the whole episode here.
If you have Films on Demand, watch the whole episode here.
If you have Classroom Video, watch the whole episode here.
Most of our videos are also available on SAFARI Montage. Just log in and do a quick search.
Don’t have any of the above? Rent or buy the Shedding Light series and/or individual programs from our Vimeo page!!
The Transcript (which can be used as a textbook)
Contents:
Part A: Introduction. Zero on the Kelvin scale starts at -273°C. But what’s so special about -273°C?
Part B: Colder and Colder: How cold can you go? The coldest temperature ever recorded on Earth is about -90°C, but the outer planets of our solar system get much much colder. However, they are still really hot compared to “absolute zero”.
Part C: Coefficient of Thermal Expansion: Different things expand by different amounts when they are heated.
Part D: Kelvins: As things get colder and colder, the atoms that make them up vibrate with less and less energy. At some point the atoms stop vibrating, so they can’t get any colder of course.
Part E: What’s So Good about the Kelvin Scale? It has allowed us to build the industries that our modern world depends on, that’s what!
Part A: Introduction
Temperature Scales! They’re hugely important of course because they allow us to express the temperature of something accurately. On a hot day, we can’t just say, it’s so hot it’s 10 outta 10. What does that even mean? If it gets even hotter, can we say 11 outta 10? We can, but it’s not helpful.
A proper temperature scale is made by deciding on two fixed, easily reproducible points and then assigning a value to these points.
The Celsius scale calls the melting point of ice 0°C and calls the boiling point of water 100°C and there are 100 equal divisions between these two temperatures. Human body temperature is about 37°C.
Anders Celsius, who first came up with the scale that now bears his name, could have called the boiling point of water 1000°C if he wanted to. If he did, human body temperature would be 370°C. However, 100°C was chosen for the boiling point of water so our body temperature is 37°C.
The Fahrenheit scale calls the melting point of ice 32°F and the boiling point of water 212°F. There are 180 equal divisions in between. Human body temperature therefore is about 99°F.
Neither of these temperature scales is better than the other. It’s just what you get used to. I live in Australia so I prefer the Celsius scale because that’s the one I’m used to. However there is a temperature scale that’s better than both of them and it’s called the Kelvin scale.
The Kelvin scale was named after Lord Kelvin, an Irish-born scientist who worked mostly in Scotland. He developed the idea for the scale in about the 1840s.
The Kelvin scale is used by scientists and engineers in a wide variety of fields, but as an example, by those who design gas pipes, gas storage tanks, and other vital industrial equipment that our modern world depends upon to operate.
Zero on the Kelvin scale starts at -273°C and increases in increments that are equal to the Celsius scale so that 1 on the Kelvin scale is -272°C, 2 on the Kelvin scale is -271°C and so on. And what’s so special about -273°C? Well, -273°C is as cold as anything can get. Nothing can get colder than -273°C.
I’ll say it again. Nothing can get colder than -273°C so it makes sense to make a temperature scale that calls the lowest temperature possible zero! You can’t go below zero on the Kelvin scale. So how do we know that nothing can get colder than -273°C, or what is called zero kelvin, and how exactly is the Kelvin scale better than any other scale? Let’s take a look.
Part B: Colder and Colder.
The temperature in this room is about 23°C (73°F). Inside a fridge it’s about 4°C, and in a freezer, it’s about -18°C.
We’ve already seen that when something is hot, its atoms are vibrating or moving quickly, but when something is cold, its atoms are vibrating or moving slowly. The average kinetic energy of the atoms or molecules that make up something determines its temperature.
Minus 18°C is pretty cold so the atoms and molecules in this freezer are moving with relatively little energy.
Dry ice (solid carbon dioxide) is -78.5°C (-109.3°F). The carbon dioxide molecules that make up the dry ice are moving with even less energy. However, dry ice is really hot compared to -273°C.
The coldest temperature ever recorded on Earth (in nature) is about -90°C in the ice-covered mountains of Antarctica, but other planets are much colder. Let’s take a quick tour of the Solar System. The centre of each yellow dot represents the relative distance that each planet orbits the sun. The sizes of the planets though are not to scale.
Generally, the further a planet is from the sun, the colder it is of course. The surface temperature of the sun is about 6,000°C but it’s much hotter in the centre. Mercury has an average temperature of about 430°C and Venus averages about 470°C. Venus is hotter (even though it’s further away) because it has a thick atmosphere which traps more heat. Earth has an average temperature of about 15°C.
The hottest place on Earth is a place called Death Valley in California, USA, where in summer its average top temperature is about 45°C (113°F). That’s just the average. It often gets much hotter.
The coldest place on Earth is Antarctica. The coast of Antarctica averages about -10°C (14°F), but inland it gets much much colder. The lowest temperature ever recorded was, as I mentioned, about -90°C (-130°F).
Back to the rest of the planets. Mars averages about -30°C, Jupiter about -110°C, Saturn about -140°C, and Uranus and Neptune both about -200°C. Pluto, which on the scale diagram would be another third of the screen you’re watching past Neptune to the right, averages about -230°C. Now remember, these are only averages. During each planet’s daytime, the temperatures are higher and of course at night the temperatures are lower.
By the way, and this has got nothing to do with our topic of heat, this is the size of the planets to scale, but the distances between the planets are not to scale. If, say, on a scale model, the sun had a diameter of 1 metre, a scale model of Earth would have a diameter of only 0.9 cm (that is, 9 mm, it’s just under 1/100 of the diameter of the sun) and it would have to be placed about 108 metres away from the sun. A scale model of Neptune would have a diameter of 3.6 cm (4 times that of the Earth) and would have to placed 3.2 kilometres away! As I said, the middle of each of the yellow dots shows the relative distances of the planets, but it’s pretty much impossible to show a scaled diagram of the whole solar system since the planets are basically tiny little specks of rock and gas in the vast, otherwise empty expanse of space.
| Scale Model Diameter | Relative Distance | |
| Sun | 1 metre | – |
| Earth | 0.9 cm (9 mm) | 108 metres |
| Neptune | 3.6 cm | 3.2 kilometres |
Anyway, back to our topic, the further you get from the sun, the colder it gets, but there’s a limit.
It turns out that the coldest temperature possible is -273°C (or if you like accuracy -273.15°C). Because of the fact you can’t colder, it’s been given a special name: absolute zero. Not approximately zero, not round about zero, but absolute zero. So how did they figure this out?
Well it has to do with expansion and contraction. As things get hotter, they expand. The liquid in a thermometer, for example, expands when it gets hot and so it rises up the tube. The amount that it rises is an indication of the temperature. The hotter it gets, the more it expands, so it shows a higher temperature. The reason things expand, as I explained in our previous episode, is that the atoms that make them up vibrate faster and faster and they push each other apart. When things get cold, they contract. The atoms slow down and get closer and closer together.
Now if atoms move (or vibrate) more and more slowly as they get colder, that implies that eventually they might stop completely and if they do stop completely that means that they can’t get any slower. Which means that the thing can’t get any colder.
So how did they work out that -273°C is as cold as it gets? They did it by measuring how much things expand and contract and working backwards. Let’s take a look.
Part C: Coefficient of Thermal Expansion
This thing that I’m heating is called a bi-metal strip. One side is made of copper and the other side is made of steel. The two metals have been welded together. Though it started straight, it began to curve as it was heated. This is because the copper side expanded more than the steel side. Different things expand by different amounts when they’re heated.
When I cooled the bi-metal strip down, both metals contracted back down to their original length and the bi-metal strip straightened up again.
A measurement called the coefficient of thermal expansion allows us to calculate how much something will expand as it heats up. The coefficient of thermal expansion of a substance is the length that a 1-metre-long piece of material will expand for every degree Celsius that its temperature changes. Let me explain.
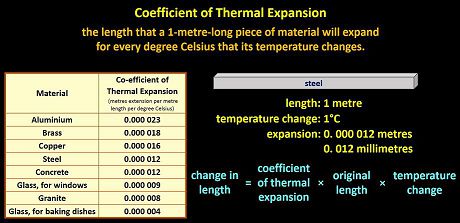Here are the coefficients of thermal expansion of various common materials.
So, for example, if you start with a 1 metre length of steel at, say, 20°C and it’s heated so that it undergoes a temperature change of 1°C, it will expand by 0.000 012 metres, which is 12 millionths of a metre, which is 0.012 millimetres. It’s barely anything at all, but that’s only a 1°C change.
The longer it is and the greater its temperature change, the more something will expand, and different substances expand by different amounts.
| Material | Co-efficient of Thermal Expansion
(metres per metre length per degree Celsius) |
| Steel | 0.000 012 |
| Aluminium | 0.000 023 |
| Brass | 0.000 018 |
| Copper | 0.000 016 |
| Granite | 0.000 008 |
| Concrete | 0.000 012 |
| Glass, Pyrex | 0.000 004 |
| Glass, plate | 0.000 009 |
We can use a simple equation to calculate the overall change in a length of material.
The change in length = Co-efficient of Thermal Expansion x the original length x the temperature change (not the temperature that it starts at or finishes at, but the temperature change).
Let’s do an example. Engineers designing a 35-metre-long concrete bridge expect the lowest temperature that it will ever reach to be -5°C and the hottest temperature it will ever reach to be 50°C. How much will it expand if its temperature changes from -5°C to +50°C?
Well, the change in length = the Co-efficient of Thermal Expansion (which is 0.000012) x the original length (which is 35 m) x the temperature change (which is 55°C) and this equates to 0.022 m, which is 2.2 cm. It’s not much but if there were no expansion gaps, the bridge would rip itself apart.
The formula is written with symbols as 
L = 
x L0 x T where
- L = the change in length (in metres) (the triangle is a delta and delta in maths and science refers to a change in a quantity)
- (pronounced alpha) = the coefficient of thermal expansion of the material (in metres per metre length per degree Celsius)
- L0 (pronounced L nought) = the original length of the material (in metres)
- T = the change in temperature (in degrees Celsius)
Some kids stress out when they see new symbols, but the formula itself is really simple.
Now concrete is rarely used on its own because it’s not actually that strong. However, concrete with steel beams running through it, which is called reinforced concrete is very very strong. You can see the steel mesh here that ends up embedded in the concrete. Structures made of concrete are actually made of reinforced concrete. Steel and concrete, luckily for us, both expand by the same amount when heated. If, in a different reality, one happened to expand more than the other, we may not have been able to build the kinds of structures that we’re familiar with. The steel embedded in the concrete would pull away from the concrete and cause it to crack, which would, over time, lead to failure. And so we’re kind of lucky that steel and concrete have the same coefficient of thermal expansion.
Part D: Kelvins
So different solids will expand and contract by measurable amounts when they heat and cool down. Gases will also expand and contract—by measurable amounts—when they heat up or cool down.
We saw in our last episode that a gas will expand when it’s heated and it will contract when it cools down. But how much do gases expand and contract?
Here I have two balloons which are the same size and which are at the same temperature.
Using some dry ice, which is at a temperature of -78.5°C, I cooled one of the balloons right down inside this insulated cooler. Ten minutes later, I placed it back next to the other one. It’s pretty obvious that the air in the cooler balloon has contracted quite a lot. Its volume, that is, the space it takes up, has decreased. Air contracts when it cools down. However within about a minute, the air warmed up again and the balloon expanded as it warmed up and went back to its original volume. Photo of the original set up, photo after being in dry-ice, photo 1 minute after it had been taken out of the dry ice. Original, Cold, Warmed back up.
Working out the amount that gases contract when they cool down was the key to calculating the coldest temperature possible.
The atoms and molecules that make up air move with a higher speed on average when they’re warmer and with a lower speed on average when they’re cooler.
The faster-moving atoms in the warmer air crash into the inside walls of the balloon with more speed and more force and push the balloon outwards. The slower moving atoms in the cooler air crash into the inside walls of the balloon with less speed and less force and so the balloon contracts inwards.
It turns out that unlike solids and liquids which all expand and contract by different amounts when they heat up or cool down, different gases all expand and contract by more or less the same amount when they heat up or cool down. But what’s this got to do with -273°C being the coldest temperature you can get? Let’s have a look at the numbers.
The Co-efficient of Thermal Expansion of solids that we saw earlier really refers to linear expansion, that is, how much the length of, for example, a 1 m long piece of steel will expand. With gases, we don’t talk about length, because gases fill up whatever container they’re in. Instead we talk about volume or pressure.
So, if you start with a certain volume of air in a balloon that is at 0°C, let’s just say 10 litres but it could be any volume, and you cool it down to -1°C, you’ll find that it has lost 1/273 of its original volume.
If you cool it down by another degree Celsius to -2°C you’ll find that it has lost another 1/273 of the original volume that it had when it was at 0°C. In other words it will have lost 2/273 of its original volume. I’ve really exaggerated the volume changes here, by the way. Cooling it down to -3°C results in the loss of another 1/273 of the volume it had originally when it was at 0°C, so now it has lost 3/273 of its original volume. When it gets down to -4°C it will have lost 4/273 of its original volume and of course this pattern continues.
On a Volume vs Temperature graph, we can see that at 0°C, the gas has a certain volume. If it loses 1/273 of this volume for every degree Celsius that its temperature falls, the line will eventually hit the x-axis—the temperature axis—at -273°C.
In the 1840s, Lord Kelvin used this information to suggest that, since the graph hits the axis at -273°C, nothing can get colder than this. And he was right!
Of course, the atoms still take up some space at -273°C—they can’t lose their volume completely and disappear, Lord Kelvin knew that—but, he correctly suggested that at -273°C the atoms can’t get any colder because they will have basically stopped moving.
He proposed a new scale that started at what was called absolute zero. This scale is now called the Kelvin scale in his honour.
By the way, Lord Kelvin’s actual or original name was William Thomson. Because of the many scientific discoveries he made throughout his life, Queen Victoria knighted him in 1866 and he became Sir William Thomson. In 1892, the British parliament made him a Lord (it’s an even higher honour than becoming a knight) and he was given the official title of Lord Kelvin after the River Kelvin that flows near the University of Glasgow where he did a lot of his work. The British system of giving honours can be pretty complicated, but let’s get back to the science!
(You’ll have to read up a little if you want to know more about how the British system of titles works! If you study the history of the British Isles, you’ll read all about kings, queens, princes, princesses, barons, earls, dukes, duchesses, lords, ladies, knights, dames and more. In 2011, a young Catherine Middleton married Prince William of England and is now officially known as Her Royal Highness, Princess Catherine, the Duchess of Cambridge.)
So, as atoms get colder and colder, they move with less and less energy. At -273°C or 0 Kelvin, atoms can’t vibrate any slower—they basically stop—so that’s as low as you can go.
Scientists don’t say degrees Kelvin like they say degrees Celsius, they simply say kelvin.
Typically, temperature is quoted in Kelvin (singular), and temperature difference is quoted in kelvins (plural), but if I was marking your test, I would probably give you full marks whether you say kelvin or kelvins.
Converting between Kelvins and degrees Celsius is really easy. You just use the simple equations:
Temperature in Kelvin = Temperature in degrees Celsius + 273 and
Temperature in degrees Celsius = Temperature in Kelvin – 273.
So, for example, what’s 20°C in kelvin? Well, using the first equation 20°C = 293 Kelvin.
And of course, in case it not obvious, a temperature difference of 1 kelvin is exactly the same as a temperature difference of 1°C.
Now more recently, it was discovered that atoms can never stop completely, they always have some energy, but William Thomson, who as I said later had a name change to Lord Kelvin, was correct that -273°C is the coldest you can get.
Part E: What’s So Good about the Kelvin Scale?
So, why is the Kelvin scale better than the other scales? It’s better because it allows scientists to apply mathematics much more easily to any processes that involve heat and temperature.
For example, if a gas is heated and allowed to expand in a flexible container its volume will increase. The percentage increase in a gas’s volume is equal to its percentage increase in temperature on the Kelvin scale.
If the temperature of say 5 litres of a gas starts at 293 Kelvin and the gas doubles in temperature to 586 Kelvin, then the volume of the gas will also double. So, the percentage increase in a gas’s volume (a 100% increase in this case) is the same as its percentage increase in its temperature in Kelvin (also a 100% increase in this case). This doesn’t work with the Celsius or the Fahrenheit scales.
If the temperature of a gas—in Kelvin—increases by, say, 20%, the volume of the gas will also increase by 20%.
By the way, I’m assuming in this example that the pressure remains the same before and after. If a gas is heated in a rigid container, the volume doesn’t increase, but the pressure does. The percentage change in pressure is also equal to the percentage change in temperature in Kelvins (as long as the volume doesn’t change). Here, for example, a doubling in temperature (from 300 Kelvin to 600 Kelvin) results in a doubling of the pressure. A 50% increase in temperature in Kelvins results in a 50% increase in pressure.
But let’s get back to volume because it’s a little easier to picture. By how much will the volume of 5 litres of gas increase if its temperature increases from 0°C to 30°C? We have to convert the temperature values to kelvin so the gas goes from 273 K to 303 K (since the temperature in Kelvin = the temperature in degrees Celsius + 273). The percentage increase = the change in temperature divided by the initial temperature times 100%. This equals 30 K/273 K times 100% which equals 11%. If the temperature in kelvins has increased by 11%, the volume of the gas will also increase by 11%.
The gas had a volume of 5 litres to begin with, so 11% of 5 litres = 11/100 times 5 litres which equals 0.55 litres. This is the increase in volume, so the final volume of the balloon after it heats up is 5.55 litres.
Now expanding balloons may seem a little unimportant, but the 
Kelvin scale is used by, for example, scientists and engineers who design oil refineries that produce our fuel and ammonia-producing plants that produce most of the world’s fertilizers for our farms, and in many other industries where gases are heated and cooled. It allows the engineers to build pipes, valves, and storage tanks that are strong enough to do their jobs. Our whole society relies on these industries to produce the things that they produce. The fuel and fertilizer industries alone are worth billions and billions of dollars.
The Kelvin scale is also used in calculating the temperature of distant stars based on the colour of light they produce and a whole lot of other things.
So, if you just want to know the weather forecast or the temperature that you need to set an oven (this one’s marked in degrees Celsius), no temperature scale is really better than any other, but a large part of our modern world is built on calculations that use the Kelvin scale.
Now so far in our series, we’ve looked at diffusion, temperature scales, the differences between solids, liquids, and gases, and at thermal expansion and all these things are related.
But, we haven’t yet looked at how heat can transfer from one object to another. How does, for example, the heat from the flame under the pot get from the flame to the water in the pot. To fully understand heat we need to understand how it transfers from one thing to another and so that’s what we’ll be looking at in our next episode. See you then.
Credits:
Written, directed, and presented by Spiro Liacos.
The simulations of the solids, liquids, and gases were created by
PhET Interactive Simulations
University of Colorado Boulder
https://phet.colorado.edu