Shedding Light on Motion Episode 8: Newton’s Third Law

The Shedding Light on Motion series is a visual treasure trove of demonstrations, animations, and explanations of all things motion! To an extent we’re all familiar with motion because we all move and we see movement everywhere, but a detailed knowledge of motion has allowed us to build the wonderful modern world that we live in.

In Episode 8, Newton’s Third Law, we look at that most poetic of all Laws: For every action there is an equal and opposite reaction. How does a rocket engine work? Why do guns recoil when they’re fired? How do our muscles work? What propels us when we’re swimming? And how exactly is gravity a two-way interaction? All these questions, and many more, will be answered in this excellent video.

A 5-minute excerpt followed by a 1-minute trailer.

The Episode 8 Question Sheet for Students:
QS8 Newton’s Third Law.
Get the answers.

If you have ClickView, watch the whole episode here.
If you have Learn360, watch the whole episode here.
If you have Films on Demand, watch the whole episode here.
If you have Classroom Video, watch the whole episode here.
Most of our videos are also available on SAFARI Montage. Just log in and do a quick search.

Don’t have any of the above? Rent or buy the Shedding Light series and/or individual programs from our Vimeo page!!

The Transcript (which can be used as a textbook)

Contents:
Part A: Introduction.
Part B: Action-Reaction Forces in Collisions. If a moving car slams into the back of stationary car, both cars experience destructive forces. If two humans collide, they will both experience a force. Forces always come in pairs called action-reaction pairs.
Part C: Moving the Earth. Newton’s Third Law doesn’t always seem to apply. But it always does. Always!
Part D: Pushing Off Each Other. If you’re on a skateboard and you push off a wall, you can propel yourself, but how does a rocket propel itself when it’s in space? Robert Goddard was ridiculed for suggesting in the 1920s that rockets would one day fly in space, but, unlike his detractors, he actually understood Newton’s Third Law, and so will you by the end of this fantastic video!

Part A: Introduction
Why do guns kick back when they’re fired? How does a rocket engine work? Why does the cue ball typically slow down when it hits a coloured ball, which speeds up? And why do I roll backwards when I throw a medicine ball forwards?
Well, the answers to all these questions involve what we call Newton’s Third Law of Motion. Newton’s Third Law of Motion is often stated as a little kind of rhyme: for every action there is an equal and opposite reaction.

What does that mean? It means that forces never, and I mean literally never, exist on their own. Newton’s Third Law says that whenever an object, let’s call it object A exerts a force on Object B, then Object B exerts an equally sized force back on Object A.

Even though it’s not always obvious, forces always come in pairs, which are called action-reaction pairs. In this lesson we’re going to look at Newton’s Third Law and at how it helps explain a range of different phenomena.

Part B: Action-Reaction Forces in Collisions

If I push this trolley, Trolley A, into this trolley, Trolley B, you can probably guess what’s going to happen. Trolley B will experience a force that will accelerate it towards the right. Let’s have a look.

No surprises there. Trolley A provided a force on trolley B which made it accelerate. However there wasn’t just one force acting, there were two. At the same time that A was exerting a force on B which made B speed up, B was exerting a force on A in the opposite direction. So what happened to A as a result of this force? Well it slowed down.

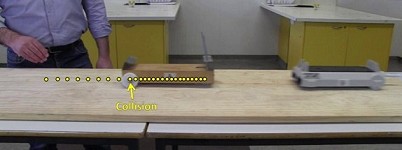
As you can see, the dots are clearly closer together after the collision. The trolley being struck doesn’t even have to do anything. Just the fact that it’s there and that a force is being exerted on it by trolley A, means that it exerts a force back on trolley A.

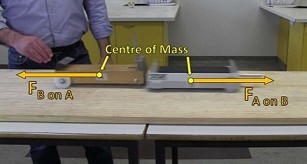
We can label the two equally sized, but opposite-in-direction action-reaction forces at the moment of impact: F_AonB and F_BonA, and as usual we’ve drawn the forces as if they’re acting on the centre of mass of the trolleys.

The same thing happens in this collision. Car A exerts a force on car B, which will make Car B accelerate, but as it does, car B exerts in the opposite direction an equally sized force on car A which makes it slow down. Of course friction is also acting but that’s a different story.

Now the expression reaction force kind of implies that it comes after the action force but in fact the two forces, which are equal in size but which act in the opposite direction to one another occur at exactly the same time.

Billiard ball collisions also involve Newton’s Third Law… The cue ball exerts a force on the coloured ball which speeds up and at the same time the coloured ball exerts a force back on the cue ball which causes it to slow down. This process can occur any number of times in quick succession. The so-called “Newton’s Cradle” demonstrates this exact principle as well.

As does this collision. Human A exerts a force on Human B so that Human B speeds up, and at the same time, Human B, just by being there, exerts a force back on Human A, which makes Human A slow down.

Now even though the action force and the reaction force are always, always, equal in size, the effect on the acceleration of each object varies depending on the mass of the objects that the forces act on.

Let’s go back to Newton’s Second Law for a moment: F_net = ma. For any given force, the acceleration of an object will be smaller when the mass is larger. If we rearrange the formula we get a = F/m. For example if a force of 1 Newton is applied to a 2 kg mass, it’s acceleration will be 0.5 m/s/s, but if a 1 Newton force is applied to a 100 kg mass, it’s acceleration will only be 0.01 m/s/s. A given force will produce a different acceleration depending on the mass that the force is being applied on.

So, I’m going to crash the trolleys together again, but this time I’m going to change the mass of the moving trolley by adding weights to it. Now just to make it a little easier to see the effect of the action/reaction pairs, I’ve also stuck some Velcro tabs on the trolleys so that they stick together when they collide.

In this collision, the Force of A on B is the same as the force of B on A, and since the trolleys are more or less the same mass, the amount that one slows down is more or less the same as the amount that the other speeds up.

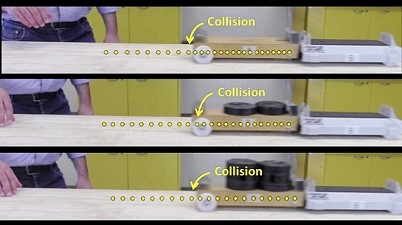When I add 2 kg to Trolley A, I have to push it with more force, but when the trolleys collide, the force that Trolley A applies to Trolley B is once again the same as the Force that Trolley B applies on Trolley A, although it’s different to the force in our first example. Since the two equally sized forces are acting on two different masses, they have different accelerations. Trolley B, being less massive, accelerates more, while Trolley A being more massive, doesn’t decelerate as much. Remember, acceleration and deceleration are kind of the same thing: deceleration is just negative acceleration.

If I now add 6 kg to Trolley A, once again, the size of the force of Trolley A on Trolley B is the same as the size of the force of Trolley B on back on Trolley A, but because Trolley A is more massive the force has less effect on it and so it hardly slows down at all. Trolley B, being far less massive has a far greater acceleration and so speeds up very quickly.

So, once again, even though the 2 forces in an action-reaction pair are always, always, equal in size, the effect of the forces in terms of acceleration will usually be different depending on the mass of the object that each of the forces acts on.

Catching a ball requires you to apply a stopping force on the ball, but while you’re applying a force on the ball, it’s applying a force on you, which can sometimes hurt. To reduce the pain, it’s better to move your hands back a little as the ball makes contact. The increased time that the stopping force is applied for results in a reduced force, meaning it doesn’t hurt as much.

Many students actually find Newton’s Third Law hard to believe. They assume that, for example, when a truck collides with a car, the force of the truck on the car (let me freeze frame the animation) is bigger than the force of the car on the truck, since the truck is so much more massive. In fact though, the forces are equal in size (but in the opposite direction to each other). Just the accelerations are different, and Sir Isaac Newton worked it all out in the 1680s, more than 400 years ago.

In this collision, the car underwent a huge change in velocity, while the truck barely slowed down at all. The same thing happened here. Because of the huge changes in velocity of a car, the occupants of a car are often injured pretty badly, if not fatally, whereas the truck drivers, who don’t change their velocity much, usually escape injury. This guy had a very bad day, but it could have much much worse.

When you kick a ball, you apply a force on the ball which accelerates it in the direction of the force, but the ball applies an equally sized force in the opposite direction on your foot which stops it swinging forward as fast as what it would have swung forward if the ball wasn’t there. It’s not really that obvious with a ball that’s doesn’t have a large mass, but when you try to kick a medicine ball, your foot’s deceleration is quite noticeable.

Of course when you’re kicking, there isn’t just one action/reaction pair. Your leg muscles are also applying a force on your foot, you’re pushing off the ground with your other leg, your arms are swinging to help you maintain balance, there’s friction which stops you from slipping, there’s air resistance; it’s pretty complicated. However the basic fact remains: whenever object A exerts a force on Object B, Object B also exerts an equal and opposite reaction force back on Object A. The less massive object will accelerate at a higher rate than the more massive object.

Part C: Moving the Earth

Now a really big problem that people often have with Newton’s Third Law is that it doesn’t always seem to apply. What happens, for example, when I drop this ball? There’s obviously a force that acts on it that pulls it downwards: it’s the gravitational force of the Earth that pulls it downwards.

Let me zoom right out, drop the ball and then freeze frame. I can label the force on the ball as F_{earth on ball}. Now Newton’s Third Law suggests that there must be an equal and opposite force that acts. What is this force? Well it’s the gravitational force that the ball exerts on the Earth which I’ll label as F_{ball on earth}. Now this is the hard bit: the two forces are exactly the same size. The gravitational force of the Earth pulling on the ball is the same size as the gravitational force of the ball pulling on the Earth. So, clearly the ball accelerates towards the Earth, but why doesn’t the Earth accelerate towards the ball. Well it does!

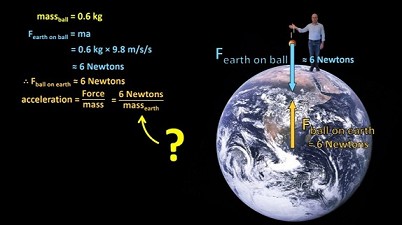The force of the Earth’s gravity acting downwards on a falling 0.6 kg ball is ma which = 0.6 kg x 9.8 m/s/s which equals about 6 Newtons. The force therefore of the ball’s gravity pulling the Earth upwards must also be 6 Newtons. So what’s the acceleration of the Earth when there’s a force of 6 Newtons on it? Acceleration = Force/mass, which in this case = 6 Newtons/m_earth. But do we know the mass of the Earth? Well, yes we do.

Scientists have actually measured the mass of the Earth by timing how long it takes certain satellites, whose height above the Earth is known precisely, to make one orbit. Using some pretty complex equations, they’ve calculated that it’s about 6 million million million million kilograms. That a 6 with 24 zeros after it. In standard form we would write 6 x 10²⁴ kg.

So back to our falling ball. What’s the acceleration of the incredibly massive Earth when a force of 6 Newtons acts on it? Well it’s basically zero but if we do the maths anyway we find that it’s only 0.000 well 23 zeros and then a 1 m/s/s (1 x 10^-24 m/s/s).

In the time that it takes the ball to fall about a metre or so, the earth would move upwards by some tiny fraction of the width of an atom’s nucleus. In other words almost zero but not quite zero. And why did I give this example? It’s just to show you that even though sometimes action/reaction pairs are not always obvious, Newton’s Third Law of Motion is still always true.

I can demonstrate kind of how gravity works by using a spring. When I stretch the spring it exerts a force back on my hands as it tries to spring back to its normal length. If I hold the spring’s left side and then stretch it to the right before letting go, it springs back towards the left. If I fix the right side in position, the spring now springs back towards the right. The spring pulls in both directions equally because of the way that the atoms are arranged in the spring.

If I extend the spring by 20 cm, the spring balance records a force of 1.8 Newtons. If I then turn the spring around and extend it the other way by the same amount, we again measure of force of 1.8 Newtons. A stretched spring pulls inwards both ways with the same force! (Different springs have different stiffnesses of course.)

The physics and chemistry of a spring is a little complicated but basically when a spring is stretched tiny groups of iron atoms get pulled apart and they try to pull themselves back together again. The force exerted by one group of atoms on another group of atoms is the same size as the force exerted by the second group of atoms back on the first.

Here I’ve stuck some small brass weights onto Trolley B so that both trolleys have an equal mass and have attached the spring between them. What happens if I pull the trolleys apart and then let go? Not surprisingly, the spring pulls the two trolleys closer together. The force pulling to the left is the same size and the force pulling towards the right, and since the masses are equal they both have the same acceleration. If I make one trolley heavier now, the spring still pulls with the same force towards the left and towards the right, but since the trolley on the left has more mass, it doesn’t accelerate as much.

If I load up Trolley A with even more mass, then it accelerates at an even slower rate. The size of force on both trolleys is the same, but Trolley A accelerates at a slower rate because it has more mass. Looking at all three together, we can see an obvious difference.

When I lift a weight up, it’s kind of like I’ve stretched an imaginary spring and then when I let go, the imaginary spring of gravity pulls the weight back down, but at the same time the imaginary spring of gravity also pulls the Earth upwards, just not to any measurable degree. So while we often think of gravity as a force that the earth applies on us, it’s really a two-way interaction between 2 objects. The 2 forces in every action-reaction pair are always the same size, but the acceleration of the two objects will be different.

Part D: Pushing Off Each Other.

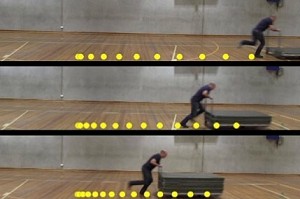
So whenever Object A exerts a force on Object B, Object B exerts a force on Object A. In this collision Object B sped up and Object A slowed down. But Newton’s Third Law of Motion doesn’t just apply when things move towards each other. It also applies when objects push off each other. If I push someone on a swing but I’m on a skateboard, she goes forward but I go backwards.

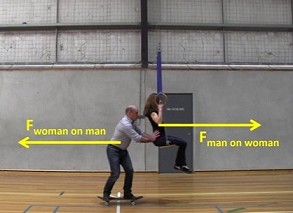
I apply a force on my wife which makes her accelerate towards the right, but she, with her presence alone, applies an equal and opposite force back on me, so I accelerate towards the left. If we both hang from wires the same thing happens. I move one way and she moves the other way. Nothing can ever accelerate without something else accelerating as well.

If I apply a force on a medicine ball, it provides a force back on me and so I move backwards. Newton’s Third Law. I don’t move as far when I push off the medicine ball because the force acts for a much short amount of time, and I don’t really build up much speed before I’m no longer in contact with the medicine ball.

Once again it’s not always obvious that there’s a backward force because you tend to lean forward when you throw and there’s usually friction between your shoes and the floor, so in fact the reaction force back on you is exerted not just on you but through you to the whole Earth, which as we’ve seen would move backwards, but just not to any measurable extent.

Let’s examine how the mass of the objects affects their accelerations. Earlier we saw how a stretched spring pulls inwards towards the left and towards the right. The same kind of thing happens with a spring that can be compressed, but it pushes outwards. If I hold the spring’s left side and then compress it to the left before letting go, it springs back towards the right. If I fix the right side in position, the spring springs back towards the left. A spring always pushes in both directions with the same sized force.

This trolley has a similar spring built into it. Since, when the spring is released, it pushes both ways, it can be used to propel either the trolley that it’s attached to, another trolley, or both.

Once again, if the two trolleys have the same mass, then their accelerations will be the same, since the atoms of the spring, which are pushing off each other, apply an equally sized force in both directions on the two equal masses. If we add mass to Trolley A, it won’t accelerate as quickly as Trolley B. Same force on both trolleys, but Trolley A accelerates at a lower rate. This becomes even more obvious the more mass we add. Here they all are at the same time.

Remember, Newton’s Third Law says that whenever Object A exerts a force on Object B, Object B will apply an equal and opposite reaction force on Object A. However, the accelerations of the two objects, that is the amount that that they speed up or slow down will be different if they have different masses.

Now muscles also, like springs, always apply a force in both directions when they contract, but it’s not always obvious. These two actions are actually almost exactly the same. Let’s look at how they work. Muscles never actually push with force, they only ever pull with force. If I want to lift up this weight, my biceps muscle shortens and pulls up on this bone along here. It’s called muscle contraction.

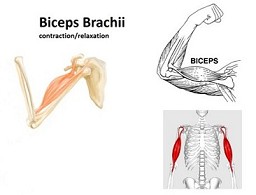Here’s a simple animation and a few images showing the biceps muscles, which technically is called the biceps brachii muscle. Now at the same time that the muscle pulls on my forearm, it also pulls on the bones that it’s attached to up here. However, since we normally anchor ourselves into position, our upper bodies don’t move much.

However, if I anchor my forearm and once again contract my biceps muscle, instead of my hand moving, my whole body is pulled towards the wall. Muscles don’t just pull one way; they pull inwards both ways.

When I extend my forearm, it’s not the biceps muscle that pushes it. Muscles can’t actually push with force. In this
position it’s gravity that pulls the weight back down as I slowly relax my biceps muscle.
In this position though, the extension of my forearm is caused by the contraction of this muscle back here called the triceps muscle, which pulls on this bone here.

The triceps contracts and extends the forearm. What we call pushing is achieved by the muscles pulling on the bones.

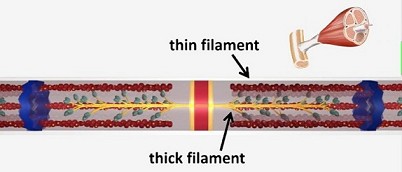Now I don’t want to get into too much detail, but basically muscles are composed of millions of fibres which are made up of individual sections called sarcomeres. These sarcomeres are made of protein filaments which are able to slide past each other. A certain part of the protein that makes up this so-called thick filament is able to latch onto the so-called thin filament and it then bends and pulls so that the filaments slide over each other. The bending involves a group of atoms pulling on another group of atoms, but of course, according to Newton’s Third Law, the second group of atoms also pulls on the first group of atoms. As a result, the muscle applies a force inwards from both ends.
When I throw a ball, a lot of the force comes from my triceps contracting. However the muscle doesn’t just apply a pulling force on my forearm, it also applies a pulling force on these bones back here. So if I anchor the ball and my forearm, but allow the rest of my body to move freely, then with exactly the same action that I threw the ball forwards, I can throw, or push, myself backwards.

Now if neither side is anchored, like when I’m on a low-friction skateboard, a throwing action results in both sides moving, because the triceps muscles are pulling both ways. My hands move forward because of my muscles contracting and I apply a force on the ball. However, the ball applies an equal and opposite reaction force on my hands which stops them from moving forward as quickly as what they would have moved forward if the ball wasn’t there. Therefore as the muscle contracts it also pulls my body away from the ball and I end up going backwards, in exactly the same way as when I pushed off the wall, although obviously not as far.

At a simple level, I apply a force on the ball which applies an equal and opposite force back on me. However there’s actually quite a few action-reaction pairs involving muscles, bones, my whole body, and the ball all happening at once. Once again, even though the point of contact is here, I’ve drawn in the force arrows from the centre of mass of the ball and my body, because the forces act on the whole of the two objects, not just at the point of contact.

Though the forces are equal in size, since the ball has less mass than I do, it accelerates at a higher rate than I do and it ends up with a higher velocity.

The same applies when I pull on this rope. Both of us here move towards each other, because my muscles are really pulling in both directions. Though the force on both of us is the same, since I have much more mass, I don’t accelerate as much. If I stand on the floor which is of course attached to the Earth, then I pull the skateboarder towards the left of screen, though in reality the whole Earth is also moving towards the right. If I now stand on the skateboard and perform exactly the same action with my muscles, then I move towards the right of screen. Once again, the whole earth is also moving towards the left of screen, but not to any measurable extent.

It’s a very similar situation when we’re swimming. Thanks to the muscles in our arms, our hands apply a force on the water, but the water applies an equal and opposite force back on our hands which stops them from moving backwards as much as what they would have moved backwards if the water wasn’t there. As a result, the contraction of our muscles results in the forward motion of our bodies. We pull ourselves along the water.

I can simulate swimming by lying on a trolley and pushing some medicine balls backwards. At a very simple level, I push on the balls and they push on me. At a slightly more advanced level, I push on the balls towards the left and they apply an equal and opposite reaction force to the right which restricts the backward motion of my hands. My muscles therefore end up pulling me forwards. It works even better when I push off heavier objects.

All of us, from a young age naturally learn to use our muscles either to move other objects or to move ourselves and so of course we don’t even think about Newton’s Third Law as we go about our daily lives. However, nothing comes naturally to a robot of course. In order for a robot to move, to overcome obstacles, and to use its arms and legs to either push something else or to push itself, robot designers have to take Newton’s Third Law into account and program the robot to, for example, lean the right way and avoid falling over when an unexpected force comes its way. The study of human movement, the application of electronics, the engineering of the robot’s motors, structure and software, and of course a good knowledge of Newton’s Laws are all very important.

Understanding Newton’s Laws of Motion is also important in the development of computer games.

Let’s see a demonstration of Newton’s Third Law in the weightless environment of the International Space Station with two of its astronauts. (Footage shows two astronauts pushing off each other and floating off in opposite directions.) Very very elegant, and certainly a lot more elegant than this Newton’s Third Law demonstration here on Earth!

The approximately 90-metre-long ISS was built in stages. Each module was lifted into orbit either by the US Space Shuttle or by various Russian rockets and then kind of bolted into place. But how do rockets work? As we’ve seen, in order for anything to be propelled, it has to push off something. Rockets work by pushing off their own fuel. Many rockets, like this one, uses hydrogen gas as their fuel which is burned in the presence of oxygen. The hydrogen and oxygen are stored in separate tanks inside the rocket.

Let’s do a quick chemistry lesson before we go any further. Hydrogen gas is made of pairs of hydrogen atoms which are bonded together, so the chemical formula of Hydrogen is actually H₂. Oxygen gas is made of pairs of oxygen atoms which are bonded together and its formula is O₂. These groups of atoms are called molecules.

I can produce hydrogen gas in a chemical reaction between magnesium metal and hydrochloric acid and then use a rubber tube to direct the hydrogen into some soapy water which traps the gas. When I throw a match in, we can see that hydrogen is flammable. When hydrogen burns, hydrogen molecules chemically react with oxygen molecule and produce H₂O molecules: H₂O is water of course.

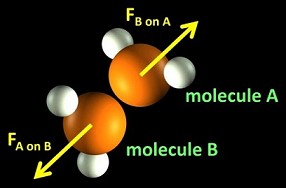Basically because of the way that the electrons move around during the chemical reaction (and electrons repel each other), the chemical reaction results in the water molecules pushing off each other at really high speeds. It’s Newton’s Third Law. (The fact that the molecules end up travelling at high speeds is what causes the temperature and pressure to rise.) Molecule A applies a force on Molecule B and at the same time Molecule B applies an equal and opposite force on Molecule A.

It’s very similar to what’s happening here. (Spiro pushing off Georgina)

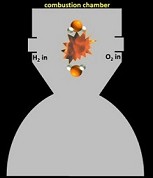Now rockets that can reach Earth orbit are very, very complicated, but basically they consist of the fuel tank and the oxygen tank, pumps, the combustion chamber where the fuel is burned (combustion means burning) and the nozzle. As I said when the hydrogen gas burns in the presence of oxygen gas, two really, really fast moving molecules of H₂O are produced which push off each other.

In the combustion chamber, one water molecule produced is pushed downwards and it exits the engine, but the other one moves upwards, remember they’re pushing off each other, and strikes the top of the engine exerting a force on it. This pushes the engine and of course the rocket upwards. It then also exits. This process is repeated again and again with trillions of molecules. The H₂O molecules produced move in every direction of course, not just conveniently up and down like we’re showing here, so looking at the bigger picture, the burning of the fuel creates a huge amount of pressure. Since there’s an opening at the bottom of the combustion chamber, there’s no pressure acting on the engine in the downwards direction. The gases moving in that direction escape the engine. The pressure acting in the opposite direction therefore pushes the rocket forward.

Basically, rockets work because as the hot gases are expelled out of the engine, they also produce an equal and opposite reaction force in the forwards direction which accelerates the rocket.

By the way, the nozzle and the so-called throat of a rocket engine improve the efficiency enormously. The throat increase the pressure and the nozzle takes advantage of the fact that the gases are still expanding as they leave the combustion chamber. The outwards pressure produces a forwards force on the angled sides of the chamber. Without these features, there would be some thrust, but not really enough to be of any use.

Though simple gunpowder-powered rockets have been around since about the 1400s, the first really powerful rockets were developed in the 1910s and 1920s by American scientist Robert Goddard. When he suggested that rockets will eventually be able to reach space, he was widely ridiculed by newspapers who asked how can rockets perform in space if they don’t have anything to push off and they accused him of not even knowing the basics of Newton’s Third Law.

But Goddard and other rocket designers had no such doubts because they knew that atoms can push off each other and create thrust. The first rocket to actually reach space was the Russian-built Sputnik which launched in 1957.

Jet engines work in a similar way to rocket engines, but rather than using oxygen stored in a tank, they burn their fuel in the presence of air which is sucked in from the front.

Balloon rockets also work in a similar way to rocket engines. A balloon is stretched when it’s full and so it provides an inwards force on the air inside it as it tries to unstretch itself to its normal size. This makes the air pressure inside the balloon higher than the air pressure outside the balloon. However, since the air pressures inside and outside the balloon are pushing equally in every direction there is no net force on the balloon. However, when the balloon is unsealed the pressure pushing on the open part is obviously not pushing on the balloon anymore. That means that the pressure of the air on the opposite side of the opening is unbalanced and so there is a net force that acts in that direction! The balloon therefore accelerates in that direction.

At a more simple level, the balloon squeezes the air out one way and so the air with an equal and opposite reaction force pushes on the balloon the other way.

And finally, why does a gun kick back when it’s fired?

Let’s look at the bullet first. A bullet which is technically called a cartridge is basically made of the bullet, the bit that gets fired out of the gun, a propellant like gunpowder which is flammable and the primer, whose job it is to ignite the propellant. When you pull the trigger, various levers and springs are moved resulting in the firing pin moving forward and striking the primer, which explodes
igniting the propellant. The expanding gases push the bullet forward.

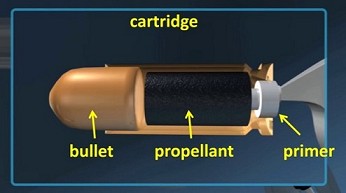However, let’s just back up a bit. As the propellant burns, which way do the expanding gases push? Well the molecules produced in the chemical reaction are pushing off each other in every direction. Newton’s Third Law. The ones that move in the direction of the bullet push the bullet down the barrel and the ones that move in the opposite direction push on the gun, which results in the recoil.

Newton’s Third Law says that the force of the gas pushing forwards on the bullet must be equal to the force of the gas pushing backwards on the gun. However since the bullet has a very small mass compared to the mass of the gun (and, of course, the person holding the gun), it has a high acceleration compared to the gun. The bullet reaches a speed of hundreds of metres per second, while the recoil speed of the gun might only be about 1 m/s.

So just recapping, Newton’s Third Law says that every time Object A exerts a force on Object B, Object B exerts an equal and opposite force back on Object A. For every action there is an equal and opposite reaction. Since forces always come in action-reaction pairs that act on two different objects, nothing can ever accelerate unless something else accelerates as well, although if you’re pushing off the earth, the earth moves so little that it’s immeasurable.
When there are lots of forces acting it’s not always easy to work out the action/reaction pairs, but they are definitely there, every single time.

And that brings us to the end of our Shedding Light on Motion series. We’ve covered speed, acceleration, relative motion, graphs and Newton’s three laws of Motion. All of these concepts have contributed to all the fantastic technology that is part of our modern world. I hope you’ve enjoyed the Shedding Light on Motion series. See you next time.

Attributions:

ATHLETICS Women’s Discus Throw Final – 28th Summer Universiade 2015 Gwangju (KOR) by FISUTV. License: Creative Commons

MilitarySkynet.com – Atlas Evolves into killer Military Robot Terminator by MilitaryClips.com. License: Creative Commons

DEADLY CRASHES, FATAL ACCIDENT, EPIC CRASHES, ULTIMATE TRUCK CRASH,, DEATH CAR by Mr. LEe. License: Creative Commons

All footage and animations of rocket launches are produced by NASA.

https://commons.wikimedia.org/wiki/File:Simple_harmonic_oscillator.gif by Oleg Alexandrov has been released into the public domain.

BASEBALL Men’s SemiFinal Match KOR vs TPE – 28th Summer Universiade 2015 Gwangju (KOR) by FISUTV. License: Creative Commons

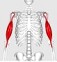File:Biceps brachii muscle01.png (https://commons.wikimedia.org/wiki/File:Biceps_brachii_muscle01.png) by en:Anatomography is licensed under the Creative Commons Attribution-Share Alike 2.1 Japan license.

File:Animation biceps.gif (https://commons.wikimedia.org/wiki/File:Animation_biceps.gif) by Niwadare is licensed under the Creative Commons Attribution-Share Alike 4.0 International license.

File:Animation triceps biceps.gif (https://commons.wikimedia.org/wiki/File:Animation_triceps_biceps.gif) by Niwadare is licensed under the Creative Commons Attribution-Share Alike 4.0 International license.

Create your own images of whatever muscles and just about every other body part at http://lifesciencedb.jp/bp3d/?lng=en. I (Spiro Liacos) “created” the image of the skeleton with the triceps muscles at their fantastic interactive website.